OMCEND

The approach developed within the framework of the project for modeling a simple armature (static cable) combines two methods. On the one hand, we use a specific two-dimensional numerical method, which preserves the analytical description of the problem in a third direction, that of propagation, which is helical. This method allows us to take into account the continuous helical symmetry of the armor and its surrounding sheaths. On the other hand, we used periodic media theory to take into account the discrete rotational symmetry of the reinforcement section. Taking these two types of symmetry into account in the models makes it possible to considerably reduce the size of the problems to be solved without making any approximations (a reduction by a factor of more than 1,000 compared to a complete 3D model). As an indication, we can successively go from a number of degrees of freedom (size of the matrices involved in the numerical solutions) of around two billion in 3D (Figure 2, left), to one million in 2D (Figure 2, right), and finally to only 20,000 per rotational symmetry (Figure 3, left). This provides access to high-frequency waves, which are necessary for any non-destructive evaluation technique using guided waves. The models take into account the effects of strand-sheath contact and viscoelastic losses in the materials.

Figure 2 : 3D model of a simple armor (left) and its 2D cross-section (right).

The simple armor model can be used to calculate both the fields associated with applied static loads (external pressure, elongation, etc.) and the dispersion curves of wave modes (wave velocity, attenuation, , etc.) – see Figure 3. The model has been validated numerically and experimentally. In particular, the simulations make it possible to estimate wave propagation distances, which is crucial for assessing the feasibility of guided wave-based NDT techniques.

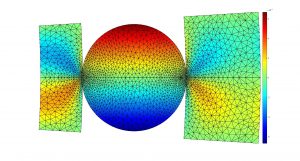
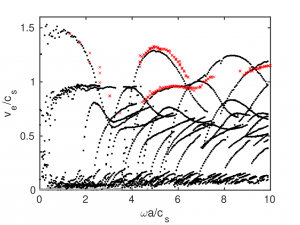
Figure 3 : Numerical results for a simple reinforcement. Left: mesh of the unit cell of the problem and microscopic field of static axial displacement. Right: normalized energy velocity curves as a function of wave mode frequency. Red crosses: experimental results.

The experimental results obtained on samples with a broken wire defect confirm the trends observed in the numerical results, namely that the most attractive modes for NDT tend to be in the low frequency range (see Figure 4). Two modes that are potentially interesting for NDT of armour wires have been identified: the L(0.3) mode around 1.3MHz, with attenuation of around 30dB (i.e. a propagation distance of 2 to 3m), and the L(0.1) mode, which is the least attenuated mode, with attenuation of around 15dB around 400kHz (i.e., a propagation distance twice as long, or 4 to 6m). The L(0.2) mode has attenuation comparable to L(0.3) but is less excitable. The higher-order modes L(0.n) (n>3) are significantly more attenuated than the others.

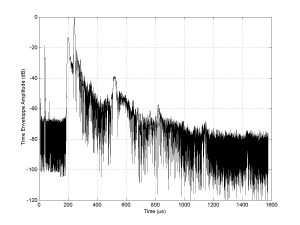
Figure 4 : Time signals measured experimentally during transmission in an umbilical wire (broadband excitation). The different peaks correspond to the echoes generated by the ends of the sample. A time-frequency analysis shows that the most echogenic modes are the low-frequency modes.

A magnetostrictive device was implemented. This device has the advantage of being contactless (locally encircling). Its operation in a low-frequency regime, which is more compatible with the propagation of the L(0,1) mode, made it possible to increase the propagation distance of this mode to 9 m at low frequency (see Figure 5). Furthermore, this device is capable of detecting a fairly small clear defect corresponding to a single broken strand out of 50 (i.e., a 2% reduction in the cross-section of the armor). It should be noted that the L(0,1) mode is a low-frequency mode, likely to behave globally, involving the dynamics of the entire cable cross-section. This can be seen as a disadvantage if the specific aim is to detect defects in the armor, or an advantage for more global NDT. The global or local nature of the L(0,1) mode at low frequency is an open question, which the work carried out as part of the project does not allow us to decide.

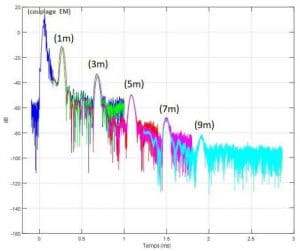
Figure 5 : Stacking of envelopes of temporal arrivals in L(0,1) mode, detected by a magnetostrictive transceiver device in a low-frequency domain.

No double armor samples were available during the project. The only results obtained for this structure are therefore numerical. The modeling approach developed for a single armor is not applicable in the case of a double armor (dynamic cable). This is because the two layers of armor rotate in opposite directions, which completely breaks the continuous symmetry of the problem. The approach we have developed is based on the theory of periodic media in two directions. For double armor, the unit cell of the problem is thus reduced to a three-dimensional cell whose dimensions are of the order of the diameter of the strands (see Figure 6 on the left). However, theoretical difficulties arise due to the curvature of the two axes of periodicity (double helix geometry). As part of the project, we proposed a specific bi-helical coordinate system, proved the existence of wave modes in such a geometry, and then established the conditions for the numerical implementation of our approach. The numerical results obtained for double weave (see Figure 6, right) show trends similar to those observed for single weave.

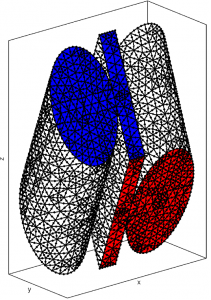
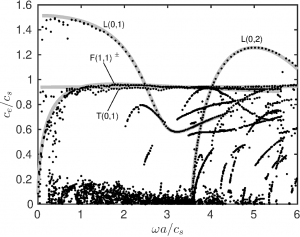
Figure 6 : Numerical results for a double armature. Left: reduction of the mesh to the repetitive unit cell of the problem; right: normalized energy velocity curves as a function of wave mode frequency (gray lines: case of a free strand).

The project results suggest that appropriate instrumentation could be used to monitor a dynamic cable. This would involve designing magnetostrictive transmit-receive collars, placed near critical damage zones (connectors, hubs, etc.) – see Figure 7. Magnetostrictive devices offer numerous advantages. No special access is required as it can be mounted directly on the outer sheath. Its relatively low-frequency operating regime allows the L(0,1) mode to propagate over a distance of around ten meters. This propagation distance appears to be compatible with the size of critical areas. This type of device is global. It does not require wire-by-wire instrumentation and is therefore less expensive and easier to implement. The expertise acquired during the project in terms of modeling and experimentation methods can be exploited for other cable architectures and other types of devices if necessary.

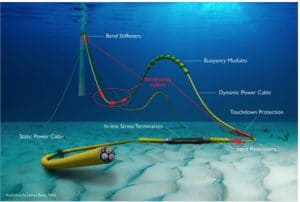
Figure 7 : Floating wind turbine cable. In red: instrumentation located around critical areas using external magnetostrictive collars.

Perspectives

Development of reliable, low-cost in-situ techniques for non-destructive testing and monitoring of cable mechanical integrity.